Differentiation is used to analyze the properties such as intervals of increase, decrease, local maximum, local minimum of quadratic functions.
A - Quadratic Function in General form
Quadratic functions in their general form are written as
\[f(x) = ax^2 + bx + c\]where \(a\), \(b\), and \(c\) are real numbers such that \(a \neq 0\).
The first derivative of \(f\) is given by
\[ f'(x) = 2ax + b \]Let us analyze the sign of \(f'\) and hence determine any maximum or minimum point and the intervals of increase and decrease. \(f'(x)\) is positive if
\[ 2ax + b > 0 \]which may be written as\[ 2 a x > - b \]We now need to consider two cases and continue solving the inequality above.
case 1: coefficient \(a > 0\)
We divide both sides of the inequality by \(2a\) and solve it to obtain
\[ x > -\frac{b}{2a} \]We now use a table to analyze the sign of \(f'\) and whether \(f\) is increasing over a given interval.

The quadratic function with \(a > 0\) has a minimum point at \((-b/2a, f(-b/2a))\) and the function is decreasing on the interval \((- \infty, -b/2a)\) and increasing over the interval \((-b/2a, + \infty)\).
case 2: coefficient \(a \lt 0\)
We divide both sides of the inequality by \(2a\) but because \(a\) is less than 0, we need to change the symbol of inequality
\[ x \lt -\frac{b}{2a} \]We now analyze the sign of \(f'\) using the table below

The quadratic function with \(a \lt 0\) has a maximum point at \((-b/2a, f(-b/2a))\) and the function is increasing on the interval \((- \infty, -b/2a)\) and decreasing over the interval \((-b/2a, + \infty)\).
B - Quadratic Function in Vertex form
Quadratic functions in their vertex form are written as
\[ f(x) = a(x - h)^2 + k \]where \(a\), \(h\), and \(k\) are real numbers with \(a \neq 0\).
The first derivative of \(f\) is given by
\[ f'(x) = 2a(x - h) \]We analyze the sign of \(f'\) using a table. \(f'(x)\) is positive if
\[ a(x - h) > 0 \]We need to consider two cases again and continue solving the inequality above.
case 1: coefficient \(a > 0\)
We divide both sides of the inequality by \(a\) and solve the inequality
\[ x > h \]The table below is used to analyze the sign of \(f'\).

The quadratic function with \(a > 0\) has a minimum at the point \((h, k)\) and it is decreasing on the interval \((- \infty, h)\) and increasing over the interval \((h, + \infty)\).
case 2: coefficient \(a \lt 0\)
We divide both sides of the inequality by \(a\) but we need to change the symbol of inequality because \(a\) is less than 0.
\[ x \lt h \]We analyze the sign of \(f'\) using the table below

The quadratic function with \(a \lt 0\) has a maximum point at \((h, k)\) and the function is increasing on the interval \((- \infty, h)\) and decreasing over the interval \((h, + \infty)\).
Example 1
Find the extremum (minimum or maximum) of the quadratic function \(f\) given by
\[ f(x) = 2x^2 - 8x + 1 \]Solution to Example 1
- We first find the derivative
\(f'(x) = 4x - 8\)
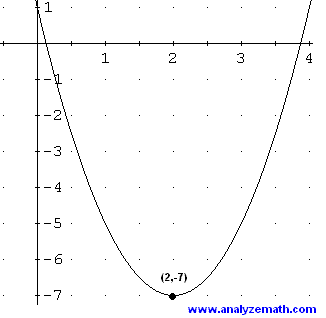
\(f'(x)\) changes sign at \(x = \frac{8}{4} = 2\). The leading coefficient \(a\) is positive hence \(f\) has a minimum at \((2, f(2)) = (2, -7)\) and \(f\) is decreasing on \((- \infty, 2)\) and increasing on \((2, + \infty)\). See graph below to confirm the result obtained by calculations.
Example 2
Find the extremum (minimum or maximum) of the quadratic function \(f\) given by
\[ f(x) = - (x + 3)^2 + 1 \]Solution to Example 2
- The derivative is given by
\(f'(x) = -2(x + 3)\)
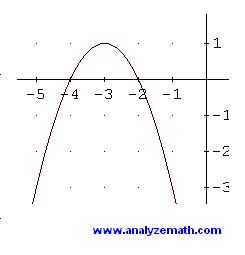
\(f'(x)\) changes sign at \(x = -3\). The leading coefficient \(a\) is negative hence \(f\) has a maximum at \((-3, 1)\) and \(f\) is increasing on \((- \infty, -3)\) and decreasing on \((-3, + \infty)\). See graph below of \(f\) below.
Exercises on Properties of Quadratic Functions
For each quadratic function below find the extremum (minimum or maximum), the interval of increase and the interval of decrease.a) \(f(x) = x^2 + 6x\)
b) \(f(x) = -x^2 - 2x + 3\)
c) \(f(x) = x^2 - 5\)
d) \(f(x) = -(x - 4)^2 + 2\)
e) \(f(x) = -x^2\)
Answers to Above Exercises
a) minimum at the point \( (-3, -9) \)decreasing on \( (-\infty, -3) \)
increasing on \( (-3, +\infty) \)
b) maximum at at the point \( (-1, 4) \)
increasing on the interval \( (-\infty, -1) \)
decreasing on the interval \( (-1, +\infty) \)
c) minimum at at the point \( (0 , -5) \)
decreasing on the interval\( (-\infty, 0) \)
increasing on the interval \( (0, +\infty) \)
d) maximum at \( (4, 2) \)
increasing on the interval \( (-\infty, 4) \)
decreasing on the interval \( (4, +\infty) \)
e) maximum at \( (0, 0) \)
increasing on the interval \( (-\infty, 0) \)
decreasing on the interval \( (0, +\infty) \)
More on applications of differentiation
applications of differentiation